Difference between revisions of "Triangular Traction Law"
| Line 10: | Line 10: | ||
<math>J_c = {1\over 2} \sigma\delta_c</math> | <math>J_c = {1\over 2} \sigma\delta_c</math> | ||
When creating this traction law, you have to enter exactly two of these failure properties for both mode and mode II (i.e., two of J<sub>Ic</sub>, σ<sub>I</sub>, and δ<sub>Ic</sub> and two of J<sub>IIc</sub>, σ<sub>II</sub>, and δ<sub>IIc</sub>). Whichever property is not specified will be calculated from the other properties using the following relations: | When creating this traction law, you have to enter exactly two of these failure properties for both mode I and mode II (i.e., two of J<sub>Ic</sub>, σ<sub>I</sub>, and δ<sub>Ic</sub> and two of J<sub>IIc</sub>, σ<sub>II</sub>, and δ<sub>IIc</sub>). Whichever property is not specified will be calculated from the other properties using the following relations: | ||
| | ||
<math>\delta_c = {J_c\over 500\sigma}, \qquad \sigma = {J_c\over 500\delta_c}, \qquad {\rm or} \qquad J_c = 500\sigma\delta_c</math> | <math>\delta_c = {J_c\over 500\sigma}, \qquad \sigma = {J_c\over 500\delta_c}, \qquad {\rm or} \qquad J_c = 500\sigma\delta_c</math> | ||
These relations assume J<sub>Ic</sub> in J/m<sup>2</ | These relations assume J<sub>Ic</sub> in J/m<sup>2</sup>, σ in MPa, and δ<sub>c</sub> in mm. | ||
To get the initial rise of the | To get the initial rise and location of the peak, you can provide, k, and the relative crack opening displacement at the peak stress, δ<sub>peak</sub> (it is relative to the critical opening displacement and therefore from 0 to 1). You must enter zero or one of these terms above for both mode I and mode II (''i.e.'', zero or one of k<sub>Ie</sub> and δ<sub>I,peak</sub> and zero or one of k<sub>IIe</sub> and δ<sub>II,peak</sub>). If one is provided, the other will be calculated from: | ||
You must enter zero or one of | |||
| |||
<math>k = :{\sigma\over \delta_{peak}\delta_c}</math> | |||
If neither k nor δ<sub>peak</sub> are entered, the peak will be located at δ<sub>peak</sub> = 0.225926299. This location provides the closest match to the [[Cubic Traction Law|cubic traction law] for area under the curve as a function of cod. The initial slope will be set to | |||
| |||
<math>k = :{\sigma\over 0.225926299 \delta_c}</math> | |||
Revision as of 23:24, 6 January 2014
Traction Law
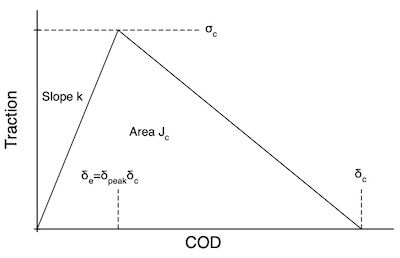
The traction laws in pure mode I or pure mode II each have the triangular shape shown in the figure. There are separate traction laws for opening displacement (mode I) and sliding displacement (mode II).
The toughness of this traction law is the area under the curve or:
[math]\displaystyle{ J_c = {1\over 2} \sigma\delta_c }[/math]
When creating this traction law, you have to enter exactly two of these failure properties for both mode I and mode II (i.e., two of JIc, σI, and δIc and two of JIIc, σII, and δIIc). Whichever property is not specified will be calculated from the other properties using the following relations:
[math]\displaystyle{ \delta_c = {J_c\over 500\sigma}, \qquad \sigma = {J_c\over 500\delta_c}, \qquad {\rm or} \qquad J_c = 500\sigma\delta_c }[/math]
These relations assume JIc in J/m2, σ in MPa, and δc in mm.
To get the initial rise and location of the peak, you can provide, k, and the relative crack opening displacement at the peak stress, δpeak (it is relative to the critical opening displacement and therefore from 0 to 1). You must enter zero or one of these terms above for both mode I and mode II (i.e., zero or one of kIe and δI,peak and zero or one of kIIe and δII,peak). If one is provided, the other will be calculated from:
[math]\displaystyle{ k = :{\sigma\over \delta_{peak}\delta_c} }[/math]
If neither k nor δpeak are entered, the peak will be located at δpeak = 0.225926299. This location provides the closest match to the [[Cubic Traction Law|cubic traction law] for area under the curve as a function of cod. The initial slope will be set to
[math]\displaystyle{ k = :{\sigma\over 0.225926299 \delta_c} }[/math]