Difference between revisions of "Coupled Traction Law"
Jump to navigation
Jump to search
(Created page with "__TOC__ == The Traction Law == right This traction law assumes the triangular shape for traction as a function of crack opending d...") |
|||
| Line 4: | Line 4: | ||
[[File:Tritraction.jpg|right]] | [[File:Tritraction.jpg|right]] | ||
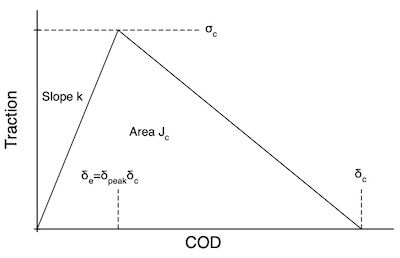
This [[Traction Laws|traction law]] | This [[Traction Laws|traction law]] is the same as the [[Triangular Traction Law|triangular traction law]] except that COD and traction are now effective terms that are related to normal and tangential components of traction and displacement: | ||
| |||
<math> {\rm Traction} = T_{eff} \qquad{\rm where} \qquad T_{eff} = \sqrt{T_n^2+T_t^2}</math> | |||
| |||
<math> {\rm cod} = \delta_{eff} \qquad{\rm where} \qquad \delta_{eff} = \sqrt{\delta_n^2+\delta_t^2}</math> | |||
where T<sub>n</sub> and T<sub>t</sub> are the normal and tangential tractions and δ<sub>n</sub> and δ<sub>t</sub> are the normal and tangential CODs. | |||
== Failure == | == Failure == | ||
Revision as of 19:38, 7 January 2014
The Traction Law
This traction law is the same as the triangular traction law except that COD and traction are now effective terms that are related to normal and tangential components of traction and displacement:
[math]\displaystyle{ {\rm Traction} = T_{eff} \qquad{\rm where} \qquad T_{eff} = \sqrt{T_n^2+T_t^2} }[/math]
[math]\displaystyle{ {\rm cod} = \delta_{eff} \qquad{\rm where} \qquad \delta_{eff} = \sqrt{\delta_n^2+\delta_t^2} }[/math]
where Tn and Tt are the normal and tangential tractions and δn and δt are the normal and tangential CODs.
Failure
Traction Law Properties
The following properties are used to create a triangular traction law:
| Property | Description | Units | Default |
|---|---|---|---|
| (other) | Properties common to all traction laws | varies | varies |