Difference between revisions of "Cubic Traction Law"
| Line 4: | Line 4: | ||
[[File:Cubictraction.jpg|right]] | [[File:Cubictraction.jpg|right]] | ||
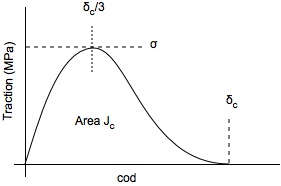
This [[Traction Laws|traction law]] assumes | This [[Traction Laws|traction law]] assumes use a cubic function for traction as a function of crack opending displacement (COD). This cubic relation is choosen such that the slope is zero at δ<sub>c</sub> and the peak always occurs at δ<sub>c</sub>. This traction law was first proposed by Needleman. It has a convenient smooth shape for numerical calculations and the zero slope at δ<sub>c</sub> may be desirable. There is no physical basis to claim it is more realistic than other traction laws. The resulting function is | ||
| |||
<math>\sigma = {27\over 4}\sigma_{max} {\delta\over\delta_c}\left(1-{\delta\over\delta_c}\right)^2</math> | |||
. | |||
There are separate traction laws for opening displacement (mode I) and sliding displacement (mode II). | |||
== Failure == | == Failure == | ||
Revision as of 13:09, 7 January 2014
The Traction Law
This traction law assumes use a cubic function for traction as a function of crack opending displacement (COD). This cubic relation is choosen such that the slope is zero at δc and the peak always occurs at δc. This traction law was first proposed by Needleman. It has a convenient smooth shape for numerical calculations and the zero slope at δc may be desirable. There is no physical basis to claim it is more realistic than other traction laws. The resulting function is
[math]\displaystyle{ \sigma = {27\over 4}\sigma_{max} {\delta\over\delta_c}\left(1-{\delta\over\delta_c}\right)^2 }[/math] . There are separate traction laws for opening displacement (mode I) and sliding displacement (mode II).
Failure
Traction Law Properties
The following properties are used to create a triangular traction law:
| Property | Description | Units | Default |
|---|---|---|---|
| (other) | Properties common to all traction laws | varies | varies |